A-level 数学/CIE/纯数学 2/代数

模函数

模函数^{[注释 1]} $|x|$ 返回 $x$ 的大小。例如， $|\!-\!3|$ 将返回 $3$ ， $|5|$ 将返回 $5$ .

模函数可以定义为 $|x|={\begin{cases}-x,&{\text{if }}x<0\\x,&{\text{if }}x\geq 0\end{cases}}$ .

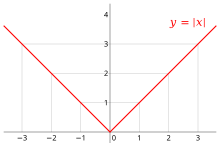
模函数的图形

模函数的图形只是直线图形，对于负输出值已反转。图形 $y=|ax+b|$ 类似于图形 $y=ax+b$ ，不同的是 x 轴下方的每个点都会向上折叠，从而形成一个 V 形图形。

此处是一个交互式图形，它显示了直线图形和该直线模图形之间的关系。

解方程和不等式

为了解涉及模函数的方程和不等式，我们可以对等式两边取平方。

例如，解 $|4x+2|>|2x-3|$

${\begin{aligned}|4x+2|&>|2x-3|\\(4x+2)^{2}&>(2x-3)^{2}\\16x^{2}+16x+4&>4x^{2}-12x+9\\12x^{2}+28x-5&>0\\x^{2}+{\frac {7}{3}}x&>{\frac {5}{12}}\\(x+{\frac {7}{6}})^{2}&>{\frac {15}{36}}+{\frac {49}{36}}\\x+{\frac {7}{6}}>{\sqrt {\frac {64}{36}}}&{\text{ or }}x+{\frac {7}{6}}<-{\sqrt {\frac {64}{36}}}\\x+{\frac {7}{6}}>{\frac {8}{6}}&{\text{ or }}x+{\frac {7}{6}}<-{\frac {8}{6}}\\x>{\frac {1}{6}}&{\text{ or }}x<-{\frac {15}{6}}\\{\text{In interval notation, }}x&\in \left(-\infty ,-{\tfrac {5}{2}}\right)\cup \left({\tfrac {1}{6}},\infty \right)\end{aligned}}$

另一种方法是观察模数内函数符号改变的位置，即 $f(x)=0$ 的位置。

$4x+2$ 在 $x=-{\frac {1}{2}}$ 处改变符号。

$2x-3$ 在 $x={\frac {3}{2}}$ 处改变符号。

${\begin{array}{ccc}{\text{Where }}x<-{\frac {1}{2}}&{\text{Where }}-\!{\frac {1}{2}}\leq x<{\frac {3}{2}}&{\text{Where }}{\frac {3}{2}}\leq x\\-(4x+2)>-(2x-3)&4x+2>-(2x-3)&4x+2>2x-3\\4x+2<2x-3&4x+2>-2x+3&4x+2>2x-3\\2x<-5&6x>1&2x>-5\\x<-{\frac {5}{2}}&x>{\frac {1}{6}}&x>-{\frac {5}{2}}\\&&{\text{Out of range}}\\\end{array}}$

${\text{In interval notation, }}x\in \left(-\infty ,-{\tfrac {5}{2}}\right)\cup \left({\tfrac {1}{6}},\infty \right)$

多项式除法

多项式除法与使用长除法进行数字除法的方法相同。

要做到
改进解释

数字除法

假设我们需要找到 $22253\div 17$ 。我们可以使用长除法的方法

  ______
17|22253

  __1___
17|22253  17 goes into 22 once with 5 left over
  -17↓    
    52    Next we bring down the 2
    
  __13__
17|22253  
  -17↓↓
    52↓   17 goes into 52 thrice with 1 left over
   -51↓
     15   Next we bring down the 5
    
  __1309
17|22253  
  -17↓↓↓  17 doesn't go into 15, so we bring down the 3
    52↓↓
   -51↓↓
     153  17 goes into 153 nine times with nothing left over
    -153
       0

因此， $22253\div 17=1309$

多项式除法

我们可以使用相同的方法来进行多项式除法。

例如， $(x^{3}+2x^{2}+2x+1)\div (x+1)$

      ____________________
x + 1 |x^3 + 2x^2 + 2x + 1

      ________x^2_________
x + 1 |x^3 + 2x^2 + 2x + 1    (x + 1) goes into (x^3 + 2x^2) x^2 times with x^2 left over
     -(x^3 +  x^2)   ↓
              x^2 + 2x         Bring down the 2x

      ________x^2_+__x____
x + 1 |x^3 + 2x^2 + 2x + 1    (x + 1) goes into (x^2 + 2x) x times with x left over
     -(x^3 +  x^2)   ↓   ↓
              x^2 + 2x   ↓     Bring down the 1
            -(x^2 +  x)  ↓
                     x + 1

      ________x^2_+__x___1
x + 1 |x^3 + 2x^2 + 2x + 1    (x + 1) goes into (x + 1) once with nothing left over
     -(x^3 +  x^2)   ↓   ↓
              x^2 + 2x   ↓     
            -(x^2 +  x)  ↓
                     x + 1
                   -(x + 1)
                         0

因此， $(x^{3}+2x^{2}+2x+1)\div (x+1)=x^{2}+x+1$

余数定理

当除数不能完全整除被除数时，就会出现余数。

例如， $22256\div 17={\frac {22253}{17}}+{\frac {3}{17}}=1309+{\frac {3}{17}}$ 余数为 $3$ 。

它也可能出现在多项式中

      ____________________
x + 2 |x^3 + 3x^2 + 3x + 3

      ________x^2_________
x + 2 |x^3 + 3x^2 + 3x + 3
     -(x^3 + 2x^2)   ↓
              x^2 + 3x
              
      ________x^2_+__x____
x + 2 |x^3 + 3x^2 + 3x + 3
     -(x^3 + 2x^2)   ↓   ↓
              x^2 + 3x   ↓
            -(x^2 + 2x)  ↓
                     x + 3

      ________x^2_+__x_+_1
x + 2 |x^3 + 3x^2 + 3x + 3
     -(x^3 + 2x^2)   ↓   ↓
              x^2 + 3x   ↓
            -(x^2 + 2x)  ↓
                     x + 3
                   -(x + 2)
                         1

这里，余数是 $1$ 。

这可以表示为 $x^{3}+3x^{2}+3x+3=(x^{2}+x+1)(x+2)+1$

一般来说，商和余数可以表示为 $Dividend=(Quotient)(Divisor)+Remainder$

这个表达式导致了数学中一个有用的定理：余数定理。

如果我们用给定的除数 $(ax-b)$ 除以多项式 $p(x)$ ，这个表达式可以写成 $p(x)=(Quotient)(ax-b)+Remainder$ .

如果我们将 $b/a$ 的值代入多项式，得到

${\begin{aligned}p(b/a)&=(Quotient)(a(b/a)-b)+Remainder\\p(b/a)&=(Quotient)(b-b)+Remainder\\p(b/a)&=(Quotient)(0)+Remainder\\p(b/a)&=Remainder\\\end{aligned}}$

因此，余数定理指出，对于给定的多项式 $p(x)$ ， $p(b/a)$ 给出了从 ${\frac {p(x)}{ax-b}}$ 获得的余数。

例如，如果 $p(x)=x^{3}+3x^{2}+3x+3$ ， $p(-2)$ 将给出从 $(x^{3}+3x^{2}+3x+3)\div (x+2)$ 获得的余数。

${\begin{aligned}p(-2)&=(-2)^{3}+3(-2)^{2}+3(-2)+3\\&=-8+3(4)-6+3\\&=-8+12-3\\&=1\end{aligned}}$

因式定理

因式定理是余数定理的一个特例，当余数为零时适用。

如果余数为零，则意味着除数是被除数的因式。

因此，如果 $p(b/a)=0$ ，则 $(ax-b)$ 是 $p(x)$ 的因式。

例如， $p(x)=x^{3}-5x^{2}+x+10$ 。使用因式定理找到 $p(x)$ 的一个因式。

${\begin{aligned}p(1)&=1^{3}-5(1^{2})+1+10=1-5+1+10=7&\neq 0\\p(-1)&=(-1)^{3}-5(-1)^{2}-1+10=-1-5-1-10=-17&\neq 0\\p(2)&=2^{3}-5(2^{2})+2+10=8-5(4)+2+10=8-20+12&=0\\\therefore \ &(x-2){\text{ is a factor of }}x^{3}-5x^{2}+x+10\end{aligned}}$

说明

↑ 也称为绝对值函数

对数和指数函数 →

[1] 也称为绝对值函数

[注释 1]